Diffractive optics are optics based on elements with operation principles which are essentially based on the phenomenon of diffraction of light. Such devices can obtain an extensive range of optical functions.
A typical aspect of diffractive optical elements is the wavelength dependence of their performance since the optical wavelength influences differences in optical phase which are essential for diffraction effects.
OpticStudio models diffractive power independent to the substrate index and the surface sag; diffractive power introduces phase change to rays. All diffractive surfaces in OpticStudio bends rays according to the following equation:
where
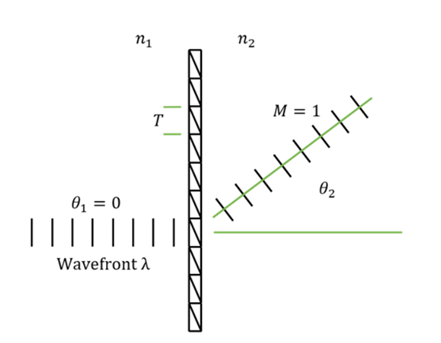
The equation above is Snell’s law for refraction, plus an additional ray-bending term representing diffraction. The diagram below shows the diffraction for a ray incident normally (sin(theta1) =0) for a diffractive surface with no refractive power.
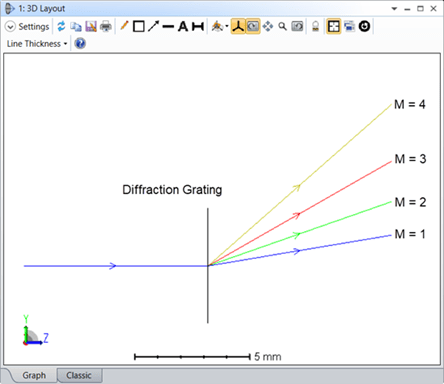
A surface such as the Diffraction Grating surface has a constant period of grating lines along one axis and is commonly used in spectrometers. The real power of computer-generated diffractive surfaces is that the grating period can vary spatially across the surface so that diffractive power can be added exactly where it is needed.
According to the equation above, the diffraction angle depends only on the period (T) of the repetitive structure where the incident light hits, and not on the shape of the structure within that particular period. The surface structure does affect the diffraction efficiency, which is not modeled by geometrical rays. The efficiency of the specified diffraction order is assumed to be 100%, meaning all rays incident on the diffractive surface will exit at the diffraction angle of the specified order.
The sign of the diffractive order determines the sign of the diffraction angle with respect to the optical axis. The sign convention for the diffraction order is purely arbitrary. The convention used by OpticStudio is positive diffraction angles (with respect to the optical axis) for positive diffraction orders.
Diffractive surfaces in OpticStudio can have refractive as well as diffractive powers. The diffractive power introduces a continuous phase across the surface, according to the formula described in the manual. Since the phase is continuous, they represent ideal diffractive optical elements (DOE), where the period of the diffractive structure is infinitesimally small or at least very small compared to the wavelength.
To maximize the diffraction efficiency in a DOE, the sag of the surface within the diffraction zones can be made such that the phase of the wavefront is parallel to the diffracted waves (of the desired diffraction order) everywhere. Figure 13.3 (b) shows a “blazed” transmission grating in which the blaze angle is optimized to maximize efficiency to a particular order [1].
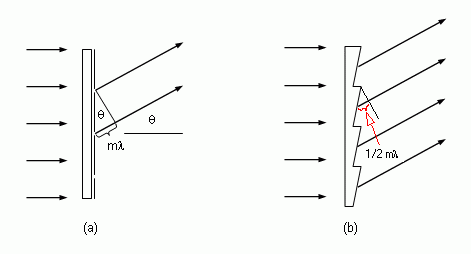
A DOE with a continuous surface profile shown in Figure (b) above is often referred to as kinoform. If the sag is approximated by discrete steps, as is often the case when photolithography is used, it is commonly referred to as a Binary Optic (see diagram below) [1]. Diffractive surfaces in OpticStudio are a closer approximation to kinoforms than true binary optics since the phase is continuous everywhere. It is up to the user to decide what surface structure to use to approximate the phase modeled by a diffractive surface.
Therefore, diffractive optics in Zemax unlock new possibilities for advanced optical system design. Leveraging Zemax’s capabilities allows designers to achieve unparalleled optical performance and system miniaturization, propelling the future of optical technology. Whether working on imaging systems, laser applications, or advanced photonics, Zemax provides the tools to effectively integrate and optimize diffractive elements, resulting in more efficient and innovative optical solutions. Examples of diffractive optics include Fiber Bragg gratings, diffraction gratings, Fresnel zone plates, and diffractive micro-optics.